1903
born December 28th in Budapest, Hungary
1913
enrolled in the Fasor Evangelical High School, his mathematics Teacher, László Rátz, discovered his mathematical talent, gave him private classes, and organized university professors to work with him
1920
won the award for Best Mathematics Student in the Country
1921-1926
completed his studies in Budapest, Berlin (chemistry), and Zurich (chemical engineering). Meanwhile, enrolled at University of Budapest with a major in mathematics and minor in physics and chemistry
1926
received a degree in chemical engineering in Zurich, and in the same year a Ph.D. in mathematics in Budapest. Went to Göttingen, and worked with Hilbert
1929
named a docent in Hamburg
1929
married Marietta Kövesi, they had a daughter, divorced in 1937
1937
received American citizenship, and became a member of the American National Academy of Sciences.
1938
married Klára Dán
1938-1957
continuously completed advisor jobs for many military institutes in the United States
1943-1945
in Los Alamos, took part in the Manhattan project (in the development of the atom bomb)
1944-1945
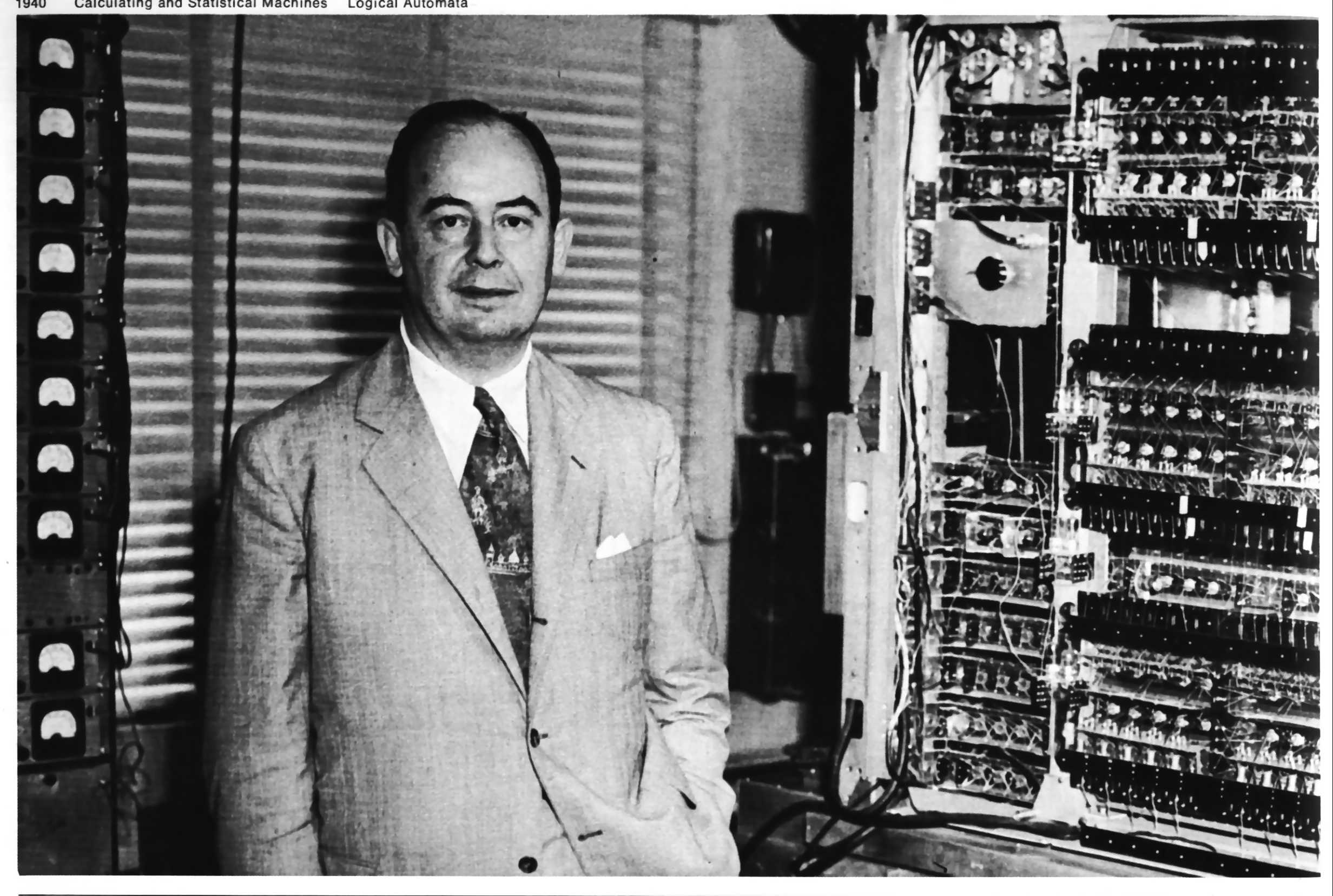
took part in the designing of the further development of the first electronic computer (ENIAC), and, generalizing the results of it, described the basic principles which define the logical structure of computers up until today
1945-1957
project director of the Electronic Computer at Princeton
1955
President Eisenhower named him a member of the American Atomic Energy Commision
1957
died February 8th from cancer in Washington, United States of America (The disease was caused by radiation sickness he got through the atom bomb research)
His father didn’t want him to be a mathematician?
To his father’s question, what he wants to be, Neumann answered mathematician. “There’s no money in that! Study something practical!” – his father answered, and took him to Tódor Kármán to talk his son out of it. Kármán wrote about it in this way in his memoirs: “In the first year of my professordom in Aachen, a Budapest banker appeared with his 17 year old son. He asked me to do a strange thing: to talk Jancsi out of becoming a mathematician. He said: ‘Mathematicians don’t know how to make money!’ – I talked to his son. He has a magnificent mind … I thought it would be a shame to shepherd him away far from where his natural inclinations lead. In Jancsi’s case, I advised his dad to make a compromise with his son: let him learn chemical engineering.” The compromise was made: he received a chemical engineering degree in Zurich, and became a Mathematics Ph.D. in Budapest.
He was legendary for his talented memory and skill at mental math?
Neumann, with his exceptional memory, actually had a photographic memory. He was able to memorize even a page from the telephone book at first sight, and he could also quote pages word-by-word from books he read long ago. When he was 6, he was already multiplying and dividing numbers with 8 digits in his head, and he checked the calculations of the first computers himself in his head, at the same time as them. The speed of his thinking was amazing.
The Neumann-priciple is a part of Hungarian heritage and his opus is a hungarikum?
Neumann made his mark as a scholar in mathematics, informatics, physics, meteorology, automation theory, game theory, and economy. In 1995, the honorable title of Hungarian Legacy was give for the first time to János Neumann and the Neumann principle. In 2015, János Neumann’s opus in the field of informatics and computers became a Hungarikum. Up until today, most info-communication tools, from desktop computers to laptops, smart phones to industrial applications, are all systems with the same development, working according to the Neumann-principle.
Neumann’s cutting in to the development of computers was a consequence of an accidental meeting?
In the early forties, Neumann’s scientific interests were mathematical description of the process of explosives (viz. Manhattan Project), which required a lot of miraculous calculations. As a consultant, he often visited the Ballistics Research Institute of the military in Aberdeen; in the summer of 1944, at the train station, he met Herman Goldstine, the leading coworker of a project in which they were building equipment with electronic circuits for the purpose of accelerating the calculations of artillery firing tables. It piqued the interest of Neumann, who soon got to know the already being built ENIAC machine, and became a participant in the working group dealing with the design of its further development.
Neumann’s professional activity can be split into two pretty distinct parts?
On the one hand, he was a world renowned scientist of his age in the “ivory tower of pure science”, and on the other, he was the “solver” of heavy practical problems, primarily for the military institutions of the United States. His daughter, Marina von Neumann-Whitman (famous economist professor, the first woman in the USA to be named an advisor to the president), writes about it in this way: “As soon as he received the American citizenship in 1937, my father … started to work for the American army, with which he cooperated until the end of his life. Even though he remained a member of the IAS up until 1955, by this time he hadn’t been engaging his brain with pure mathematics for a long time. Leaving the peaceful university environment behind him, and much to the shock of his mathematics colleagues, he changed track to the strictly confidential field of military research.”
Mathematics, physics, quantum mechanics
Neumann made his mark as a scholar in almost all the fields of mathematics. From his more than 150 essays, 20 are physics, 60 theoretical, and 60 applied mathematical studies. His career as a mathematician started with a Rockefeller scholarship in Göttingen (1926), researching operator theory and quantum physics. He soon became a university professor of the Friedrich Wilhelm University in Berlin (1927), and became an internationally known and acknowledged mathematician with his essays in set theory, quantum mechanics, and algebra. In 1929, he received an invitation to Princeton, and between 1930-1933 taught one semester in America, and one semester in Europe. In 1933, because of the coming to power of fascism, he finally settled in America. From 1933 up until his death, he was a mathematics professor of the Institution of Higher Education. In 1927, he published his famous essay on the problem of non-contradiction of mathematics. In 1932, his book, Mathematical Foundations of Quantum Mechanics, was published, which is up until the today the most foundational discussion of quantum physics. From 1940, his interest turned to the problems and research of applied mathematics (ballistics, hydrodynamics, game theory, numerical meteorology, computer theory).
Manhattan project
From 1937, he dealt with military technology research, ballistics, and hydrodynamic questions. In 1943 he joined the Manhattan Project as the advisor of the Los Alamos Laboratory, and in the period between 1943-45, he spent a significant amount of time there. The Manhattan Project was the strictly confidential program of development of the atomic weapon. Neumann’s main task was to solve the mathematical problems that came up during the development of the atom bomb. He calculated when the bomb filled with plutonium needs to be activated in the interest of achieving the desired effect so that the load would not disperse due to a premature chain reaction. After the war, Neumman was the strategic advisor to President Eisenhower in the nuclear arms race. In 1955, he was named a member to the Atomic Energy Commission, a position he fulfilled up until his death. The five Hungarian scientists working on the atom bomb (Janos Neumann, Leó Szilárd, Tódor Kármán, Jenő Wigner, Ede Teller) believed that the with power comes responsibility too. They participated in the working out of the atom bomb, although Leó Szilárd later opposed its deployment. But Neumann believed in the advantage of the pre-emptive strike, moreover, later, also in the principle of deterrence too. According to him, we can also prepare even for the worst possibilities, and that’s why he was a believer in the continuation of experimental explosives.
Economy – game theory, minimax – principle
Neumann’s work in game theory has an excessive importance. He summarized and further developed his theory in his book written together with Oskar Morgenstern in 1944, titled Theory of Games and Economic Behavior. In their work, they were modeling conflict situations. They expressed with mathematical formulas what happens when people (companies or governments) make decisions in conflict situations, what influence their thoughts about the what the other party will do and what the other party’s tactics will be have on the development of their own strategy. Analyzing zero sum two person games, they detected that the optimal strategy for both parties exists, which is the Minimax-principle. Neumann assumed that their theory would be usable in military as well as economic decision making. In 1994, its economic importance was acknowledged, and the researchers (among them János Harsányi), were awarded the Nobel Prize. The principles laid down by Neumann are still used today not only in the fields economy, social and military sciences, but pedagogy and psychology too.
Informatics – the Neumann principle and the IAS computer
In his 1945 research report, Neumann published the basic principles necessary for the ideal operation of the computer. According to the “Neumann-principle”: the computer must be completely electronic, have separate operating and executing units, use a binary number system, needs to store the data and programs in the same inner storage, the memory. For its ideal operation, the machine needs to be built from five basic functional units: input unit, memory, arithmetic unit, operating unit, output unit. Neumann published the principle, preventing anyone from taking a patent out on it. He believed that the computer is not the proper of one or more persons, but of all of humankind. It wasn’t invented by one man, but by a group of mathematicians and engineers. With his leadership, the IAS, or Neumann-machine, was built in the Institute of Higher Education in 1952. Besides the significant results achieved in the general theory of automata, he worked up until his last minute on his book titled: The Computer and Brain, uncovering the parallels between the functioning of the computer and the human brain.
Numerical meteorology
Numerical meteorology, based on real weather data, applies mathematical models to forecast local or global weather conditions. As an effect of the new scientific results, in the 1920’s the new method of atmospheric modeling was born, a partial differential equation system. Thus, the theoretical was born, then the first computer in the world, the ENIAC – more than twenty years later – created a tool so the calculations, which needed months previously, could be done in days, possibly in hours. János Neumann recommended using the ENIAC to serve exclusively military purposes, for the running of mathematical models forecasting the weather too. With his leadership, from 1946, 20 meteorologists worked on developing an atmospheric model. It was 1950 before it was ready, and they could run the first meteorological program. In their program, they tried to forecast the air flow conditions of a layer of air at a height of 5500 meters 24 hours in advance. The experiment stretched out for months due to malfunctions and frequent outages, but the clear calculation time became 24 hours. The results of the successful experiment contributed to the perfection of the equation system that was the base of the modeling.
1903.12.28 – 1957.2.8